In science, there isn’t really any such thing as a “fact”. Just different degrees of how strongly the evidence supports a theory. But diversification is about as close as we get. Closer even than evolution or gravity. In “fact”, neither evolution or gravity would work if diversification didn’t.
So I’ve been puzzled at some people’s reaction to RSCM‘s startup investing strategy. They don’t seem to truly believe in diversification. I can’t tell if they believe it intellectually but not emotionally or rather they think there is some substantial uncertainty about whether it works.
In either case, here’s my attempt at making the truth of diversification viscerally clear. It starts with a question:
Suppose I offered you a choice between the following two options:
(a) You give me $1M today and I give you $3M with certainty in 4 years.
(b) You give me $1M today and we roll a standard six-sided die. If it comes up a 6, I give you $20M in 4 years. Otherwise, you lose the $1M.
Option (b) has a slightly higher expected value of $3.33M, but an 83.33% chance of total loss. Given the literature on risk preference and loss aversion (again, I highly recommend Kahneman’s book as an introduction), I’m quite sure the vast majority of people will chose (a). There may be some individuals, enterprises, or funds who are wealthy enough that a $1M loss doesn’t bother them. In those cases, I would restate the offer. Instead of $1M, use $X where $X = 50% of total wealth. Faced with an 83.33% chance of losing 50% of their wealth, even the richest player will almost certainly chose (a).
Moreover, if I took (a) off the table and offered (b) or nothing, I’m reasonably certain that almost everyone would choose nothing. There just aren’t very many people willing to risk a substantial chance of losing half their wealth. On the other hand, if I walked up to people and credibly guaranteed I’d triple their money in 4 years, almost everyone with any spare wealth would jump at the deal.
Through diversification, you can turn option (b) into option (a).
This “trick” doesn’t require fancy math. I’ve seen people object to diversification because it relies on Modern Portfolio Theory or assumes rational actors. Not true. There is no fancy math and no questionable assumptions. In fact, any high school algebra student with a working knowledge of Excel can easily demonstrate the results.
Avoiding Total Loss
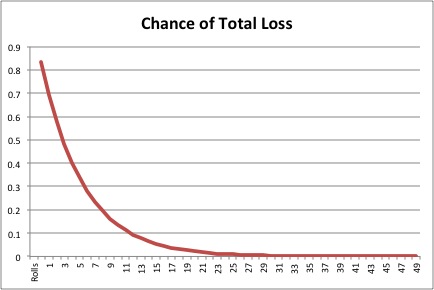
Let’s start with the goal of avoiding a total loss. As Kahneman and Tversky showed, people really don’t like the prospect of losing large amounts. If you roll the die once, your chance of total loss is (5/6) = .83. If you roll it twice, it’s (5/6)^2 = .69. Roll it ten times, it’s (5/6)^10 = .16. The following graph shows how the chance of total loss rapidly approaches zero as the number of rolls increases.
By the time you get to 50 rolls, the chance of total loss is about 1 in 10,000. By 100 rolls, it’s about 1 in 100,000,000. For comparison, the chance of being struck by lightning during those same four years is approximately 1 in 200,000 (based on the NOAA’s estimate of an annual probability of 1 in 775,000).
Tripling Your Money
Avoiding a total loss is a great step, but our ultimate question is how close can you get to a guaranteed tripling of your money. Luckily, there’s an easy way to calculate the probability of getting at least a certain number of 6s using the Binomial Theorem (which has been understood for hundreds of years). One of many online calculator’s is here. I used the BINOMDIST function of Excel in my spreadsheet.
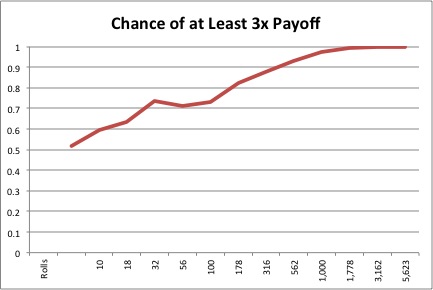
The next graph shows the probability of getting back at least 3x your money for different numbers of rolls. The horizontal axis is logarithmic, with each tick representing 1/4 of a power of 10.
As you can see, diversification can make tripling your money a near certainty. At 1,000 rolls, your probability of at least tripling up is 93%. And with that many rolls, Excel can’t even calculate the probability of getting back less than your original investment. It’s too small. At 10,000 rolls, the probability of less than tripling your money is 1 in 365,000.
So if you have the opportunity to make legitimate high-risk, high-return investments, your first question should be how to diversify. All other concerns are very secondary.
Now, I will admit that this explanation is not the last word. Our model assumes independent, identical bets with zero transaction costs. If I have time and there’s interest, I’ll address these issues in future posts. But I’m not sweeping them under the rug. I’m truly not aware of any argument that their practical effect would be significant with regards to startup investments.

4 Comments